There is a description page 9. If this architecture is used as a model for an AWES, the centifugal force would be relatively low when it scales up. Disruptive Innovation
IMHO the major feature is the tether-blade alignment allowing to minimize the cantilever effect that is encountered on current prototypes of tethered aircrafts with turbines aloft. If an AWES configuration is possible and works, this feature could be a key for scaling.
There are still more informations (see metrics page 17) on
With wind force the CSR is shaped like a cone and perhaps the aerodynamic forces on the blades don’t tend to expand the rotor (?). But it is not sure: a deeper analysis is required.
So a rotor with a bridle and undergoing… cantilever effect, looks to remain a possibility.
There are similarities to the systems discussed in this thread:
Especially having a stiff lever in the center which is turned by pulling from a kite.
In contrast to a propeller, a yoyo system like kiteswarms wouldn’t actually need the lever. It’s only needed if one wants to transfer a moment to the hub.
Hi @rschmehl ,
As you evoke Mark D. Moore on List of organizations , I opened a topic on Centrifugally Stiffened Rotor (CSR) as a model for an AWES? .
Indeed his purpose was “to put the entire structure in tension”. That can eliminate the cantilever effect of wing, and saving mass.
The CSR (blades + tethers in alignment) becomes a cone shaped due to the wind forces and I am not sure the aerodynamic forces on the blades will expand the rotor as a wing undergoing cantilever effect will do.
I would like to know your advice for this. Thanks.
In Moore’s CSR each blade is aligned with its tether.
Don’t think so. Might look like this, but to transmit moment from hub to blades, levers have to be ahead and behind to transmit moment from blades to hub. Might only be slightly. Else there is no force component on the lever tether connection point tangential to the circle in which is moves.
In the initial CSR the thrust is provided by the propellers that are settled in the end on the tips of blades. So here the hub doesn’t transmit any moment as it can be stationary as well as rotating (see page12: Central Hub Payload) with the CSR.
The centrifugal force allows to align tethers and their respective blades, saving mass.
Please read also the page 14 about bending moments of current wings compared to CSR.
The problem occurs when the CSR works as an AWES, undergoing wind force. I don’t know if it is still possible to align blade and tether, using aerodynamic force instead of centrifugal force as it scales. If yes it can be a possible winner.
Some @Windy_Skies’ comments can be related to the mode of operation of the CSR, particularly about the tether-blade alignment:
https://forum.awesystems.info/t/bladetips-energy/1615/6
https://forum.awesystems.info/t/bladetips-energy/1615/9
See the video attached to the last comment.
I think that in AWE transposition, the wind force will tilt any CSR disk loading, in addition to transform the disk plan into a cone which becomes narrow as wind force increases (as for a faster and faster rotation resulting in an increase of the lift, as explained in the comments above).
I would like to add a calculation related to this and Bladetips Energy design.
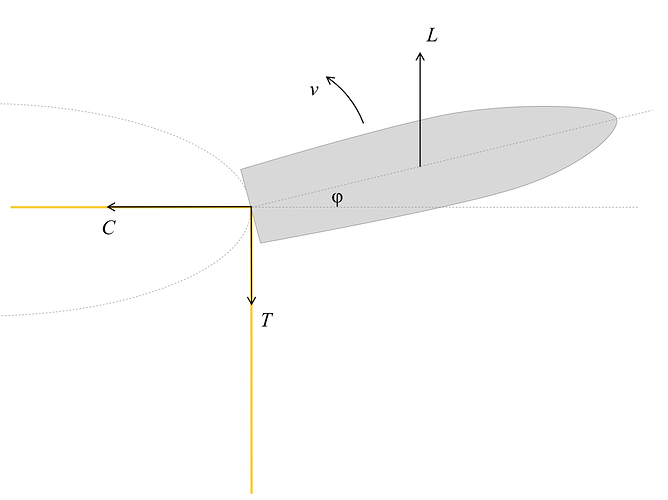
The [poor] sketch shows a rotating blade attached only at the inner end. Not shown is the looping radius R distance from center of rotation to CG [center of gravity] of the blade. C is the force towards center supported by a tether. T is the tether force going to the ground, perpendicular to the plane of rotation. Note T and C may be a bridle going somewhere in between these, but the calculations should be the same. The angle \phi is the “cone” angle; how much the blade must rotate in order to maintain balance of moments. We assume \phi is small for now. The mass of the blade is m. The blade is moving at speed v. I also assume the effect of gravity is much smaller than other forces involved here, and so it is neglected.
The force T is the one used to generate energy, as this is a bounding type AWE system with pulling energy transfer. C is balanced by other blades also rotating.
As \phi is small, we can assume T \approx L.
Balance of force due to rotation gives us C = m \frac{v^2}{R}
Lift of the wing is L = \frac{1}{2} \rho v^2 C_L S (including here density of air, lift coefficient and surface area of the wing)
The interesting part here is the balance of moments that decides the angle \phi. We have
C \sin \phi = T cos \phi
\tan \phi = \frac{T}{C}
\tan \phi = \frac{\frac{1}{2} \rho v^2 C_L S}{m \frac{v^2}{R}}
\tan \phi = \frac{ \rho C_L S R}{2 m}
At this point, we could make an assumed angle for an imagined wing with mass 15 kg, span 7 m, area 3 m2, looping radius 20 m, C_L of 1.5 and \rho 1.225. This yields
\phi \approx 75^\circ
So this is just not feasible. The thing here is you need the mass to be as big as possible to generate centrifugal forces, but this is at odds with general AWE goals. So it is a bad idea it seems to me. The other way to lower the angle \phi is to reduce the looping radius. This leads to aerodynamical problems, as the inner part of the blade will be moving more slowly. Anyways, the lowest angle I get is 32^\circ which is still very high. Note the assumtion of small \phi does not hold.
Anyways, lets see how this scales. We will assume every dimension scaled by x. Wing area scales by x^2 and mass by x^3. The angle of the scaled wing would be
\tan \phi_x = \frac{ \rho C_L \left(x^2 S\right) \left(x R\right)}{2 \left(x^3 m\right)}
\tan \phi_x = \frac{ \rho C_L S R}{2 m}
We see that the tilt angle of the blade does not change with scale, so going bigger or smaller will not help the situation.
So hereby I claim the method of attaching the blades at only one end for AWE DEBUNKED, at least for the way I understand it should be implemented.
Lets just fasten the tether for T somewhere close to center of lift…
This is draft comment from July 2022 I didn’t submit:
I liked this answer down the page (partial quote):
A linked video from above answer of an rc plane flying circles connected to a tether: Art’s RTP plane - YouTube
From 4:55: control by two tethers fixed to the cord of the inner wing and acting the elevator of the stabilizer (if I saw correctly). See also the controlled flight at about 6:10, then the full video. Perhaps some ideas for The Pyramid or some kinds of CSR?
Control line flying works with only one input because the wings are constrained to be in line with the lines. In the Pyramid, there is a single tether attachment point, so there the wings aren’t constrained like that.
I also don’t think ground control via tethers would work on a rotary torque transfer system if you have multiple kites on a line, and there is “triangular” bridling between kites at the same height, like the Daisy and Pyramid. If we assume the lines make a helix while torque transfer is happening, some of the kites would be on the bottom while others would be on top of the shaft, so they need different control inputs, while you only can send the same input up the line, “elevator down” for example.
And then there is the input lag of using control line input to kites on long lines, and mushy control through lines less than perfectly taut.
But you can of course fly (a train of) crosswind kites using ground control.
I guess it could be made to work. The Pyramid would require two control axes for roll and pitch. That means three tethers. And tension would probably have to be equal on all three tethers to avoid different performance in different windspeeds (slack tethers get much shorter than taught ones).
Im not sure how that would pan out though.
Pitch control is very sensitive. It makes sense to me to measure pitch onboard and then control it locally. Tether control seems harder for me, if you want to proceed further than a flying prototype. The challenge is open though for anyone who would like to try. I am not saying its a dead end
