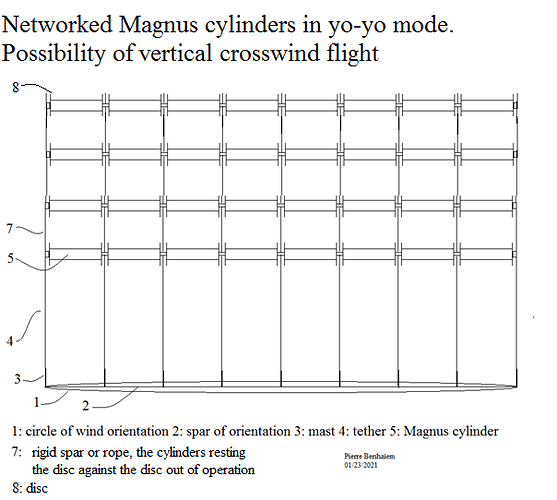
Scaling in numbers is interesting to mitigate the square-cube law. But it is not easy to put numerous small unities close each other, due to the tethers then risk of tangle, above all if unities work crosswind.
There were many discussions about (Magnus effect based) Flettner rotors. Limits of inflatable Magnus cylinders lead to a high power consumption even at a low spin ratio (below 1) where the coefficient of lift (CL 2 at the best) is far to be optimized.
However things are likely different with rigid cylinders (CL above 5, and a far lesser power consumption) which are not likely to widen under the effect of the wind. Some data are mentioned on the pdf that is available on:
https://repository.tudelft.nl/islandora/object/uuid%3Aae200352-5d8b-4551-900b-3d316cc5fd49
As a result small unities could be realized in order to avoid a too much weight penalty. thanks to cylinder topology, an unlimited number of cylinders could be put side by side or/and stacked. As the control is mainly rpm control there is no issues of turn and also of depower (in yo-yo mode, as shown by Omnidea balloon).
An example of scaling by number of Flettner rotors is given below: